Ducting Pressure Losses

HRSG designers utilize ducting for many purposes in a system design. They are used for connecting flue gas plenums to stacks, distributing combustion air to burners, transfering flue gas to the HRSG or for bypassing the HRSG. The pressure losses through ducting pieces may be individually analyzed or the may be analyzed as a system.

We will first explore ducting losses by looking at the individual pieces. The following formulas and coefficients are from the American Petroleum Institute Practice API RP533.

---

Straight duct run friction loss:
D_p = (0.002989 * F_r * r_g * V_g²)*L_e/D_e Where,

Dp = Pressure drop, inH2O

Fr = Moody friction factor

rg = Average gas density, lb/ft3

Vg = Velocity of gas, ft/sec

Le = Equivalent length of piece, ft

De = Equivalent diameter of piece, ft

And for round duct,
D_e = Diameter And for rectangular duct,
D_e = (2 * Width * Height)/(Width + Height) Moody friction factor, F_r

We can use the Colebrook equation to solve for the friction factor, with the roughness factor selected from the following:

Duct surface	Roughness
Very rough	0.01
Medium rough	0.003
Smooth	0.0005

Reynolds number:	Roughness:
	Friction factor:

---

90° Round section elbow loss:

	Dp = Vh * Cl
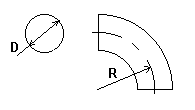Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Radius/Diameter(R/D)	Coefficient(Cl)
0.5	0.90
1.0	0.33
1.5	0.24
2.0	0.19

---

90° Rectangular section elbow loss:

		Dp = Vh * Cl
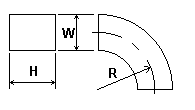Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Height/Width(H/W)	Radius/Width(R/W)	Coefficient(Cl)
0.25	0.5	1.25
	1.0	0.37
	1.5	0.19
0.50	0.5	1.10
	1.0	0.28
	1.5	0.13
1.00	0.5	1.00
	1.0	0.22
	1.5	0.09
4.0	0.5	0.96
	1.0	0.19
	1.5	0.07

---

Elbow of any degree turn loss:

This may be used for a rectangular or round duct elbow of N ° turn.

	Dp = Vh * C90 * N/90
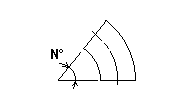Where,
Vh = Velocity head of gas, inH2O
C90 = Loss coefficient from above for 90° turn
N = Number of degrees of turn

---

Sudden contraction loss:

	Dp = Vh * Cl
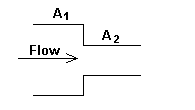Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Area2/Area1(A2/A1)	Coefficient(Cl)
< 0.2	0.34
0.2	0.32
0.4	0.25
0.6	0.16
0.8	0.06

---

Gradual contraction loss:

	Dp = Vh * Cl
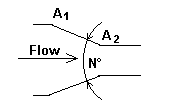Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Included Angle(N°)	Coefficient(Cl)
30	0.02
45	0.04
60	0.07

---

No contraction change of axis loss:

	Dp = Vh * Cl
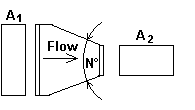Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Included Angle(N°)	Coefficient(Cl)
<=14	0.15

---

Sudden enlargement loss:

	Dp = Vh * Cl
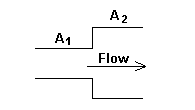Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Area1/Area2(A1/A2)	Coefficient(Cl)
0.1	0.81
0.3	0.49
0.6	0.16
0.9	0.01

---

Gradual enlargement loss:

	Dp = Vh * Cl
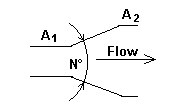Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Included Angle(N°)	Coefficient(Cl)
5	0.17
10	0.28
20	0.45
30	0.59
40	0.73

---

Sudden exit loss:

	Dp = Vh * Cl
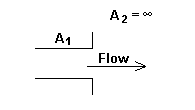Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Area1/Area2(A1/A2)	Coefficient(Cl)
0	1.0

---

90° Round miter elbow loss:

	Dp = Vh * Cl
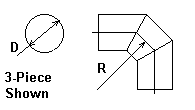Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Curve

---

90° Rectangular miter elbow loss:

	Dp = Vh * Cl
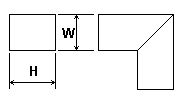Where,
Vh = Velocity head of gas, inH2O
Cl = Loss Coefficient From Table
Height/Width(H/W)	Coefficient(Cl)
0.25	1.25
0.5	1.47
1.0	1.50
4.0	1.35

---

Pressure loss across stack damper:

This pressure loss is normally accounted for by rule of thumb. This may be 0.5 or 0.25 velocity head. We will use 0.25.

D_p = 0.25 * V_h Where,

Dp = Pressure drop, inH2O

Vh = Average velocity head of stack, inH2O

---

Draft gain or loss:

The draft gain or loss will be taken based on the height of the upward or downward flow of the flue gas. If the flow is upward, the pressure loss is negative.

D_p = (r_a - r_g)/5.2 * A Where,

Dp = Draft gain or loss, inH2O

rg = Density of flue gas, lb/ft3

ra = Density of ambient air, lb/ft3

A = Height of gas path, ft

---

Velocity head of gas:
V_h = V_g² * r_g / 2 / 32.2 / 144 * 27.67783 Where,

Vg = Velocity of flue gas, ft/sec

rg = Density of flue gas, lb/ft3

---

Now we can try some of these piece calculations,

Duct Piece Type: Straight duct run Elbow Miter Elbow Sudden contraction Gradual contraction No contraction change of axis Sudden enlargement Gradual enlargement Sudden exit Stack damper Draft gain or loss	Shape: Round Rectangular
Inlet Diameter, ft:	Outlet Diameter, ft:
Inlet Width, ft:	Inlet Height, ft:
Outlet Width, ft:	Outlet Height, ft:
Radius Of Turn, ft:	Incl'd Or Turn Angle, Deg:
Roughness Factor:	Hght Of Col Or Lgth Of Run, ft:
No. Of Miter Pieces:
Gas Flow, lb/hr:	Viscosity Of Gas, cp:
Density Of Flue Gas, lb/ft3:	Density Of Ambient Air, lb/ft3:
Velocity, ft/sec:	Velocity Head, inH2O:
Coefficient:	Pressure Drop, inH2O:

Now that we have some procedures for calculating the pressure loss for the various components that we might find in a duct system, how do we use them? The easiest way to analyze a ducting system and keep the pressure points straight, is to organize the system starting from the outlet and proceeding to the inlet. This may seem backwards at first, but when you examine the pressure at a given point in the system, you find that the pressure is always dependent on the downstream pressure. So, it makes sense to always work from outlet to inlet, then you always know the pressure of the outlet of the point you are at. To try this out, we will run the calculations for the simple example shown below.

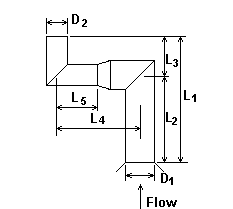For this example, we will assume we are picking up the flow at some point in a system, so we will calculate from the outlet(assumed to be to atmosphere) to the inlet without considering any condition at the inlet. We will assume the process conditions are those in above table.

So assuming,
D1 = 4 ft dia.	D2 = 3 ft dia.
L1 = 10 ft	L2 = 7 ft
L3 = 3 ft	L4 = 7 ft
L5 = 3 ft

Desctription	DeltaP, inH2O	Static, inH2O	Dynamic, inH2O	Total, inH2O
Outlet Condition	0	0	0	0
Sudden Exit	1.1242	0	1.1242	1.1242
Straight Duct Run, 2 ft	0.0136	0.0136	1.1242	1.1378
Miter Elbow, 2 Pc	1.4614	1.4750	1.1242	2.5992
Straight Duct Run, 3 ft	0.0204	1.4954	1.1242	2.6196
Gradual Contraction, 45°	0.0450	2.3089	0.3557	2.6646
Miter Elbow, 2 Pc	0.4624	2.7713	0.3557	3.1270
Straight Duct Run, 5 ft	0.0082	2.7795	0.3557	3.1352